Yuriy Rozhdestvenskiy, D.Sc. in Physics and Mathematics and professor at ITMO’s Faculty of Photonics and Optical Information, as well as young scientists Semyon Rudyi and Tatyana Vovk published an article on signature identification based on the fractal Minkowski–Bouligand dimension and methods of machine learning.
“We consider a signature as a trajectory of a pen tip that obeys the Langevin equations (stochastic differential equations that describe Brownian motion). For a signature considered in such a way we can calculate the fractal Minkowski–Bouligand dimension. This parameter differs for authentic and intentionally altered signatures, which makes it possible to reliably discern similar signatures left by different people,” explains Tatyana Vovk.
Together with machine learning techniques, this approach offers a potentially powerful tool for identification and verification of signatures and any other kind of notations. According to Yuriy Rozhdestvenskiy, machine learning is necessary for identifying similar signatures and discerning those that have different shapes. In its turn, the fractal Minkowski–Bouligand dimension allows to reliably identify whether a signature has been forged, while machine learning often fails to perform such a task.

The Minkowski–Bouligand dimension
The Minkowski–Bouligand dimension, or box-counting dimension, comes from chaotic dynamics (a branch of physics). When explaining the box-counting dimension, physicists often use the example of different curves, such as the Koch curve. Let’s say you have a line segment that is divided into three equal parts, and you replace the middle part with two segments that are equal to it. Thus, you get a curve that consists of four equal segments. At the next stage, you do the same thing with each of the four segments, and get a curve made up of 16 segments. After an infinite number of repetitions, you get a curve made up of an infinite number of segments. No matter how you scale it, the result is always the same.
This type of structures is called a fractal. You can see them everywhere: for example, in the structure of trees and their leaves and roots, as well as in crystals. What’s more, you can infinitely divide a triangle into smaller triangles, which also makes it a fractal structure.
“There’s the so-called “coastline paradox”, when it’s impossible to precisely measure the length of a coastline: the lesser is the scale (or the rod graduation of a ruler that we use to measure the coastline), the bigger its length will be. Just imagine that you want to measure the coastline of an island. When using different tools, like a rope, or a millimeter, centimeter, or meter ruler, we’ll be getting different results. The Minkowski–Bouligand dimension is what will be its characteristic length, the limit of all fragmentations”, explains Semyon Rudyi.

In case of identifying whether a signature has been forged or not, you have to take the authentic signature and measure its fractal dimension in order to compare it with the one you want to verify. If it is a forged signature, the fractal dimensions will differ.
“You can draw a smooth curve, for example a circle on a computer, and calculate its fractal dimension, which will always be the same regardless of the size and thickness of the line. Still, when two different people draw a circle each, their fractal dimensions will be different. Why? It’s simple – we are all different. When a machine identifies a person’s signature, it doesn’t account for one’s personality, what they were thinking about and how they held their hand. Fractal dimensions don’t “think” about these things either, but they were developed for describing physical stochastic systems (a stochastic process is a process which behavior is not determined, and such a system’s condition is described with both values that can be predicted and those that can’t – Ed.). This is exactly the reason why fractal dimension is most adequate to our physical reality. A person’s signature is a mixture of both random and determined factors, and our task is to identify the determined ones, while the random ones are not important,” comments Yuriy Rozhdestvenskiy.
How to calculate a fractal dimension
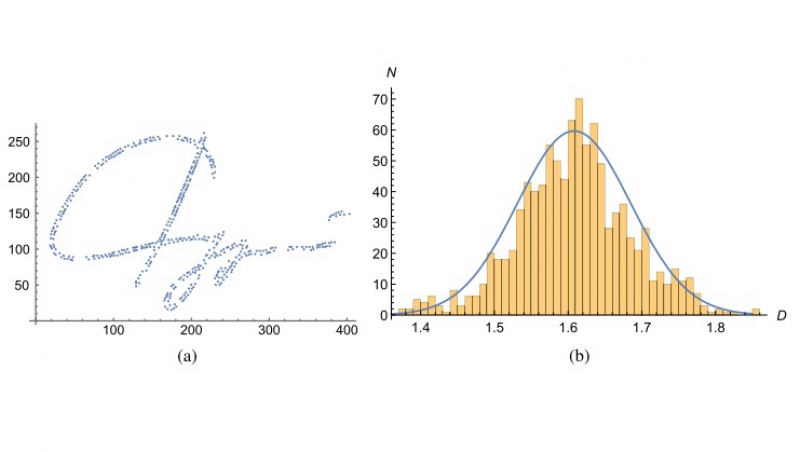
Researchers consider any signature as a trajectory of some particle that moves according to chaotic dynamics’ equations. The analysis of such a trajectory is conducted via digitalization of the signature by means of Poincare section. For this purpose, researchers take points of the signature at particular periods of time. The assembly of these points on a plane is a two-dimensional Poincare map. Then, the digitized signature is analysed by means of fractal dimension. The plane with a set of points is split into four equal squares. The squares that still contain points are also split in four lesser ones. This procedure is repeated until the number of squares that still contain points stays the same. And this parameter is what defines the box-cutting dimension.

“We use a Poincare section on the signature, which is not a complex procedure. You can identify the following axes of a trajectory: reference axis, speed axis, and time axis. When using a Poincare section, the signature trajectory is split along the speed axis by equal time intervals. The resulting assembly of points is applied over the plane of reference and speed, which is made up from the corresponding axes, and the number of points increases. The resulting set of points is analyzed by applying a grid of identical squares. We count the squares and choose the ones that contain at least one point, and record this data, the ratio of ‘occupied’ squares to the squares’ overall number. Then we increase the number of squares by four, follow the same procedure, and record the result. We do that until there’s only one point per any ‘occupied’ square, as there’s no use to go on: the number of ‘occupied’ squares will remain the same. This limit of the ratio of squares is what is called the fractal dimension. For such a task, we do 20-30 scalings. If we automate the process, it would take minutes. This is another of our invention’s benefits, as the issue of verifying a signature is always a question of time,” says Yuriy Rozhdestvenskiy.
Today’s approach to verifying signatures
A signature is a unique and important biometric marker of a person. From the times of yore, it has been used as a means of personal identification. Even now, when we have automated means for verifying signatures, in most cases experts verify signatures manually. As a rule, forged signatures are identified by means of a graphological examination conducted by forensic scientists.
“In essence, forensic scientists compare two texts by eye, focusing on how the letters are written. Then again, a person can sign differently at different times of the year, or even times of the day. It would’ve been good to have an objective tool that offered precise criteria for identifying a forgery. There’s also the issue of identifying signatures on art pieces. Such tests can only be conducted by experts. Knowing the prices in this field, you can’t be 100% sure in the tests’ quality, even considering an expert’s reputation: there’s always the human factor that we have to account for,” comments Yuriy Rozhdestvenskiy.
Other applications
Among the possible applications of the method is not just verification of individuals’ signatures, but also examination of art pieces for forgery. While the former implies working with signatures only, the latter also has to do with analyzing the curves of images, which can help tell whether a painting belongs to a particular author or not.
“A signature is a curve. A painting is a lot of curves. In this case, we can apply the same method: take the outlines of curves of a painting, analyze them and calculate their fractal dimension. Then we take a different picture the authorship of which we want to identify. Point is, a painter’s hand and sight move according to a particular pattern, they see reality in a particular manner, and when they paint, the way they draw lines is not random but has a particular logic to it. According to our hypothesis, this logic remains the same regardless of a painting’s subject, so it can be used to identify authorship,” concludes Yuriy Rozhdestvenskiy.





